Kepler: The Volume of a Wine Barrel

Kepler's Era

Johannes Kepler (1571 – 1630) was a German mathematician, astronomer, and astrologer, and a key figure in the 17th century scientific revolution. He lived after Copernicus and supported the heliocentric model of the universe. Kepler worked with Tycho Brahe and used Brahe's remarkable observational data to make his most famous discovery, the three laws of planetary motion now known as Kepler's Laws. Newton later showed that Kepler's laws could be deduced from Newton's laws of motion and universal gravitation law.

As a mathematician Kepler discovered two new regular polyhedra, worked on the problem of close packing of equal spheres, was interested in logarithms and found volumes of solids of revolution.

Our main purpose here is to understand Kepler's contributions to the development of calculus.

Kepler lived before Newton and Leibniz. During the sixteenth century and early seventeenth century, the Greek mathematical masterworks, such us Euclid's Elements, the Conics of Apollonius or the works of Archimedes, were studied seriously. Numerous mathematicians refined the method of exhaustion and applied it to a wide variety of new quadrature (area) and cubature (volume) problems. Another point of interest was the determination of centers of gravity of solids (see Margaret Baron's book p. 90 about the importance of centers of gravity for the development of the calculus). Renaissance mathematicians were more interested in new results and methods of discovery than in rigorous proofs. They freely used intuitive concepts of the infinite to produce infinitesimal methods for the solution of area and volume problems. Kepler and Cavalieri were two key mathematicians who helped invent these infinitesimal methods.

The wine barrel incident

Kepler had several children before his first wife died. In 1613, he married for the second time in a celebration in Linz, Austria. Kepler bought a barrel of wine for the wedding but questioned the method the wine merchant used to measure the volume of the barrel and thus determine the price.

In consequence, afterwards Kepler set out both to determine the correct volume of a wine barrel or cask, and to find the proportions that optimize the wolume of a wine cask.

According to Otto Toeplitz (p.83):"Working out finer approximations of various barrel shapes, he consulted Archimedes and discovered that his own method of indivisibles had enabled him to obtain results in a far simpler and more general way than Archimedes, who had been struggling with cumbersome and difficult proofs. What he did not suspect was that Archimedes, too, had found his results by the same method of indivisibles (for the Method was lost until 1906!)."

Kepler's Nova stereometria doliorum vinariorum

Kepler reported his results on wine barrels in his 1615 book, Nova stereometria doliorum vinariorum (New solid geometry of wine barrels). The word Stereometria is from the Ancient Greek stereos that means solid or three-dimensional and metron that means a measure or to measure. Then Stereometria means the art of measuring volumes or solid geometry. From the title, we use Doliometry that is an old-fashioned word from the Latin dolium that means a large jar or barrel.

This book is a systematic work on the calculation of areas and volumes by infinitesimal techniques. Building on the work of Archimedes, it focuses on solids of revolution and includes calculations of exact or approximate volumes of over ninety such solids (Edwards, p. 102). Today we use integral calculus to solve these kinds of problems.

Similarly, by thinking of the sphere as being composed of an infinite number of infinitesimal cones whose vertices were the center of the sphere and whose bases made up the surface of the sphere, Kepler was able to demonstrate that the volume of the sphere equals one-third the product of the surface area and the radius. (Recall that the volume of a cone is one-third the product of the area of its base and its height or altitude.)

Kepler also rotated a circle about a line external to the circle, and calculated, by infinitesimal methods, the volume of the torus thus generated. He then extended his work to solids not treated by the ancients. Some of his summations are remarkable anticipations of results found later in integral calculus.

Kepler in Mathworld: Lemon Kepler in Mathworld: Apple In Mathworld we can see several solids of revolution defined by Kepler.

A Problem of Maxima: Wine Barrel Design

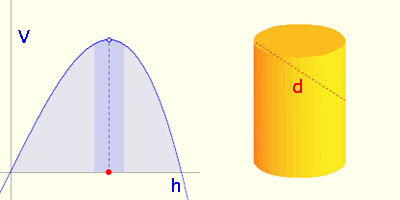
The method the wine merchant had used to measure the volume of the barrel that had so shocked Kepler was to insert a stick through the tap hole (at S in the diagram below) to the opposite edge of the lid of the barrel (at D).

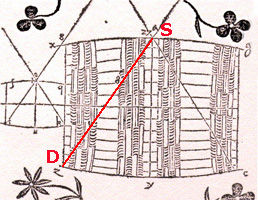
"Then he read off the length SD = d and set the price accordingly. This outraged Kepler who saw that a narrow, high barrel might have the same SD as a wide one and would indicate the same wine price, though its volume would be ever so much smaller" (Toeplitz, pp. 82-83).

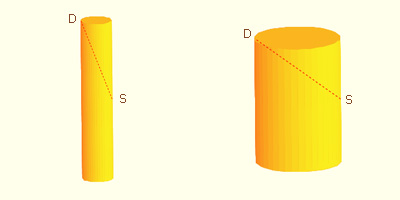
In consequence, Kepler tried to determine the best proportions for a wine barrel in order to maximize the volume. This brought him to the consideration of a number of problems on maxima and minima, which proved to be a very interesting contribution toward the development of differential calculus. For example, Kepler was able to establish that the cube is the greatest parallelepiped which can be inscribed in a sphere. (Baron, 115)

Of course, the problem of finding maxima and minima was not new. For example, Euclid had proven that among all rectangles of equal perimeter the square has the largest area (Toeplitz, 80). Pappus of Alexandria around 300 CE showed that, of all equilateral and equiangular plane figures having an equal perimeter, the figure with the greater number of angles has the greater area, and the largest such area is that of the circle with that same perimeter".

The Sagacity of Circles: A History of the Isoperimetric Problem Article by Jennifer Wiegert in Convergence. A summary of the history of the problem of finding the region of greatest area bounded by a given perimeter.

Thinking Outside the Box -- or Maybe Just About the Box Article by David Meel and Thomas Hern in Convergence. They study a more complex and realistic box problem than the traditional one and show how it can be used to investigate optimization.

To optimize the volume of the barrel, Kepler simplified the problem. He approximated the barrel by a cylinder with the diagonal measurement d as above, r the radius of the base, and h the height. In modern notation, the formula for the volume V of a cylinder is:

Then, a relation between d, h and r can be written using the Pythagorean Theorem:

Now solve for r squared:

Hence, we can deduce the formula of the volume as a function of h and d:

For fixed d, the following animation shows how the volume V changes when we modify the height h of the barrel.

Next, Kepler asked: "If d is fixed, what value of h gives the largest volume V?" (Toeplitz, p. 83). After making some calculations and comparing them, he decided the answer was:

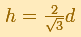
"That defined a barrel of definite proportions. Kepler noticed that in his Rhenish homeland barrels were narrower and higher than in Austria, where their shape was peculiarly close to that having a maximum volume for a fixed d - so close, indeed, that Kepler could not believe this to be accidental. So he imagined that centuries ago somebody had calculated barrel shapes, as he himself was doing, and had taught the Austrians to construct their barrels in this particular fashion - a very practical one, indeed. Kepler showed that if a barrel did not satisfy the exact mathematical specification

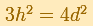
but deviated somewhat from it, this would have but little effect on the volume, because near its maximum a function changes only slowly" (Toeplitz, p. 83), as illustrated in the graph below.

Kepler tabulated values obtained by calculation to reinforce his idea that the volumes of such cylinders with equal diagonals change very little in the neighbourhood of a maximum. (Baron, p. 116)

Derivatives, Tangents and Slopes

Fermat (born in 1601) had a different approach: In modern terms, he was interested in the tangent to a curve and the relation between this tangent and the maximum (or minimum) of a function. He was the first to solve maximum-minimum problems by taking into account the characteristic behavior of a function near its extreme values.

Fermat's algebraic approach to this problem can be seen today as equivalent to studying the slope of the tangent to the curve representing the function. Newton and Leibniz had a clearer idea and they realized that a maximum or minimum was associated with a horizontal tangent.

Using our modern terminology, this is the geometric interpretation of the derivative of a function. We can see intuitively that if f(x) is a maximum (or minimum) value of the differentiable function f, then the value of f changes very slowly near x. Moreover, at the highest and lowest points on the graph of f the tangent is horizontal; that is, its slope is 0. The derivative will be zero at extrema.

Returning to Kepler's problem of the proportions of a wine barrel, if V is the volume of the barrel (as a cylinder) with a fixed value of d, then V is a polynomial in h; hence the derivative is easy to calculate:

For V to be a maximum, V' must equal zero; hence

And this was the result that Kepler found.

In the diagram below, the blue curve is the graph of y = V(h) and the red curve the graph of y = V'(h) for a fixed value of d.

Thus, the practical problem of measuring the volume of a wine barrel inspired Kepler to make important contributions to the development of both the integral and differential calculus.

References

Margaret E. Baron, The Origins of the infinitesimal Calculus, Dover Publications, New York, 1987.

Carl B. Boyer, The History of the Calculus and its Conceptual Development, Dover Publications, New York, 1959.

Links

	Kepler in Mac Tutor History of Mathematics A biography of Kepler.
	Original latin version in The Posner Memorial Collection (full book) Original german version in Convergence (two pages) Original versions of Nova Stereometria available on the web.
	Kepler in MatematicasVisuales - The Area of a Circle Kepler used an intuitive infinitesimal approach to calculate the area of a circle.
	Kepler in Mathworld: Lemon Kepler in Mathworld: Apple In Mathworld we can see several solids of revolution defined by Kepler.
	Archimedes' Method for Computing Areas and Volumes Article by Gabriela Sanchis in Loci Convergence where she explains Archimedes' Method based on the Law of the Lever.
	Archimedes in MatematicasVisuales - Area of a parabolic segment Archimedes used infinitesimal methods to discover areas an volumes 1800 years before Kepler or Cavalieri.
	The Sagacity of Circles: A History of the Isoperimetric Problem Article by Jennifer Wiegert in Convergence. A summary of the history of the problem of finding the region of greatest area bounded by a given perimeter.
	Thinking Outside the Box -- or Maybe Just About the Box Article by David Meel and Thomas Hern in Mathdl. They study a more complex and realistic box problem than the traditional one and show how it can be used to investigate optimization.

About the Author

Acknowledgements

I would like to thank Janet Beery, Convergence editor, for her support and encouragement. Without her help this article would not have been published.

I thank the anonymous referees for suggestions that improved the article.

I am grateful to the Posner Library for granting permission to use images from its copy of Kepler's Nova stereometria doliorum vinariorum in this article.

Miguel Cardil helped me in the artistic design of the page and has also designed all graphic elements in the mathlets.

I would like to thank Kathleen Killorin for her help in the English translation.